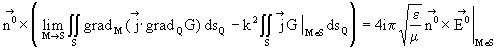|
The base of EDEM is formed by algorithms which were
obtained as the result of development of the principles suggested by the
authors for solving the problems under consideration. The detailed
description of this technique one may be found in [1,2].
In the current version of the program
algorithms for solving the six types of problems are realized. These are
plane two-dimensional problems for two kinds of polarization, a problems
for surfaces of revolution with axisymmetric excitation;
three-dimensional problems for structures of arbitrary shape, and also a
problems for surfaces of revolution in which the incident field can be
presented by quickly converging Fourier series by azimuthal coordinate.
The original problem is formulated as rigorous integral equations of
first kind for surface current density induced on the analyzed structure
by incident electromagnetic field. In case of three-dimensional problem
the integral equation is
| |
|
Tasks of other five classes are reduced to
the one-dimensional integral equations or systems of such equations
which have been written by a contour of cross-section of analyzed
surfaces.
Kernels of the equations by the special representation for scalar
potential are transformed to a form allowing subsequently greatly
to reduce quantity of calculations. As a result of the numerical
solution of the equations there are components of the currents induced
on researched structure. Then various characteristics of the
electromagnetic fields resulting from these currents are calculated
For the numerical solution it can be used both constant
approximation, and the basic functions of higher order known as RWG functions.
For the solving of systems of linear algebraic equations
both direct and iterative methods are realized, in particular
the Generalized Minimal Residual Algorithm (GMREZ).
Besides the solving of the rigorous integrated
equations, an approximate solving of tasks on the basis of a method
of physical optics is possible. The sizes of researched
structures can be at this very large.
-
Doklady
Akademii Nauk SSSR, 1984, v.276, N 1, p.96-100.
-
Journal of Communications Technology and
Electronics, Vol.45, Suppl.2, 2000, pp.S247-S259.
|